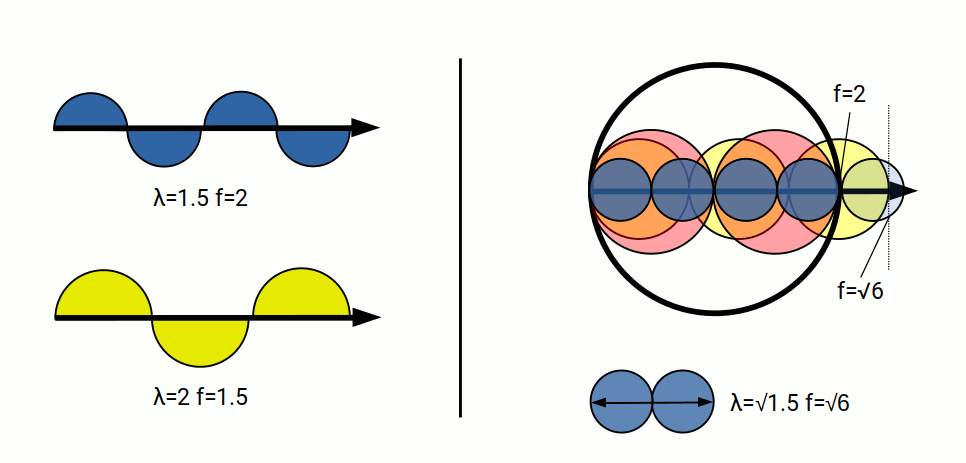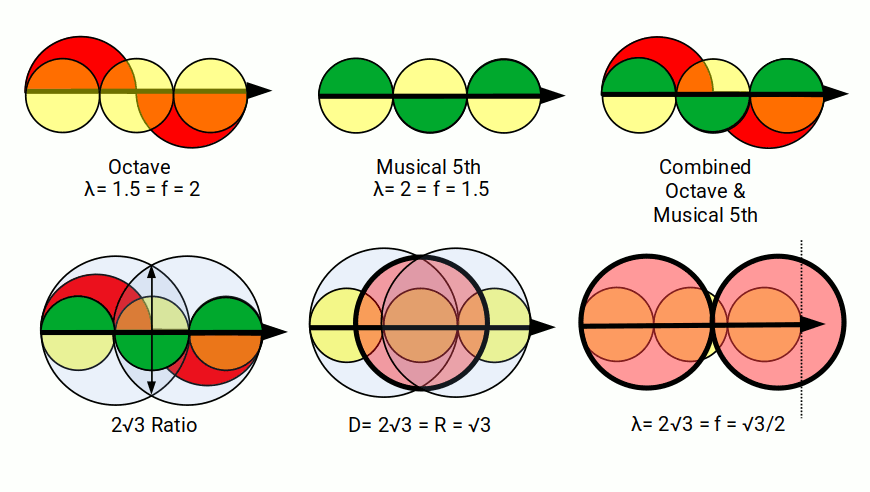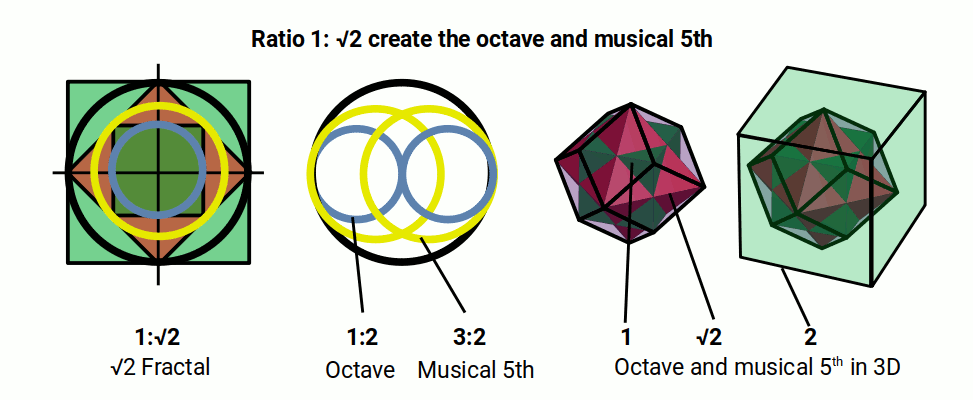When certain frequencies of light, particularly those in the ultraviolet range, are projected onto different materials, electrons are emitted from the surface. This phenomenon is known as the Photoelectric Effect. What appears to initiate this, is the frequency of light, and not its intensity, i.e. energy. This fact, along with the failings of classical wave theory to predict the spectral radiance of a Black Body, led Einstein to first postulate that light was a photon of light, transmitted in discrete packets. However, could there be an alternative explanation?
Overview
The photoelectric effect is a phenomenon in which electrically charged particles are released from a material when it absorbs electromagnetic radiation, which generates a current in a connected circuit. The fact that this phenomenon requires light above a certain frequency seemed to contradict the classical interpretation of electromagnetic waves, as it was believed that a continuous low frequency wave should build up charge over time.
When Max Plank first resolved the Ultraviolet Catastrophe by the reformulation of classical electromagnetism, his solution introduced a new constant into physics. The Planck constant (h) was a fixed unit of energy, which suggested energy was quantized at the microscopic scales of reality. Albert Einstein then used this idea of quantization to propose a solution that light existed as discrete packets of energy, photons. As lower frequencies generate photon packets with less energy than required, increasing the intensity of light will only produce more photons, each of which would be unable to initiate the photoelectric effect. Only photons of a specific frequency, each of which possesses a greater amount of energy, could initiate the emission of an electron, which is why the photoelectric effect appears to be frequency dependent.
The notion reintroduced the particle nature of light. However, as the wavelike nature of light has also been undoubtably established, this led to a peculiar paradox. Light seemed to act as both a wave and a particle. This notion of wave-particle duality now stands at the foundations of modern quantum mechanics, that first introduced the notion of probabilist mathematics into the realm of scientific exploration. Yet, the exact mechanism whereby light can exhibit both wave and particle-like qualities still remains one of the greatest mysteries facing quantum theory.
However, there are a few problems with the photon interpretation of the photoelectric effect. Most prominently, it is the fact that the effect is instantaneous. The mainstream view suggests that light carries the energy that is then transferred to the electron. However, this view does not support experimental measurements. Quite simply, if light energy is the reason, there should be a slight delay in the emission as the transfer of the electron requires a certain amount of time. Instead, we find that it is almost instantaneous, suggesting that the energy is already present within the material. Therefore, light seems to only act as a trigger to initiate the effect.
Whilst the Planck constant is used in the equations to justify the photoelectric effect, we also find that this quantity is not needed in order to express the relationship between the material and the incident light source. The relationship can be quite easily established by multiplying the work function (W), by the minimum wavelength (λ) of light that initiates the photoelectric effect. When squared, the result produces a value of around half the speed of light (c).
(Wλ)² = c/2
Furthermore, we find that light wavelength of √150 nm will produce an electromagnetic (EM) wave with a photon energy of around 10 eV. In this article, we explain the detailed reason for this, and in doing so, resolve the wave-particle duality paradox by way of simple ratio, and unify this phenomenon with the laws of 4D electromagnetism, and the qualities of the Cosmic Microwave Background.
KEy Points
-
The laws of electromagnetism operate on the same theory as musical ratio, not harmonic series, which resolves the Planck constant
-
The photoelectric effect is derived from the interation of the frequency of light and the 4D Aether at the surface level of the material
-
Energy is therefore extracted from the vacuum through resonance
the Photoelectric effect Experiment
There are a few different types of experimental setup that can be used to examine the photoelectric effect. The simplest involves cleaning the particular surface, and placing it on an electroscope. Then, using a static electrically charged rod, the plate can be made negatively charged through the process of induction. Now, more electrons are deposited onto the plate and the electroscope will show a repulsion between two metal leafs. By shining a normal incandescent light onto the plate, there will be no noticeable change in charge. However, when the light is replaced with a UV light, then immediately we see a discharge of photoelectrons, and the two leafs stop repelling each other. This video shows a great example of this simple experiment.
This is the simplest example of the photoelectric effect. More elaborate experimental setups allow us to examine the phenomena in more detail. By using a circuit connected to a power source, the plate can be charged with a positive and negative voltage. The intensity of the light can also be varied, along with the frequency. This allows for much greater measurement between the interaction of light and the electron flow. Today, these experimental results have been encoded into simple scientific simulations, that emulate these experiments. Before reading on, it is a good idea to play around with one of these simulators, so that you can gain an insight into this interesting phenomena.
The intensity of light
The first law of the photoelectric effect was discovered by Aleksandr Stoletov, who demonstrated a proportional relationship between the intensity of light and the induced current. The term intensity is a quantity in physics that basically refers to the brightness of light. We experience this everyday in terms of a dimmer switch used in household lighting. When rotating the dial, we can dim the lights by applying more resistance to the circuit. In the photoelectric effect, once the threshold frequency is reached, the intensity of light can be increased, which results in more amps flowing through the circuit.
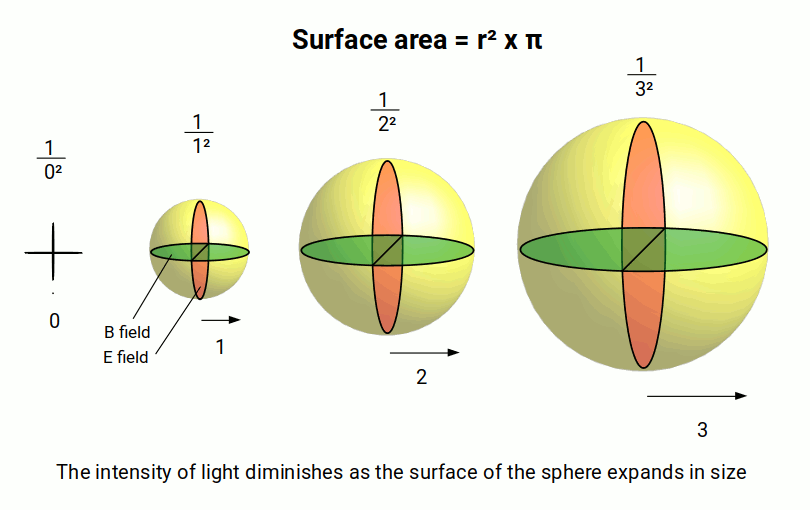
Intensity is also related to the distance of a light source by the inverse of the square. A light source can be seen to produce a spherical electromagnetic wave, that, as it expands in space, diminishes in intensity. As the equation that defines the surface area of the sphere is r² x 4π, a single unit of the surface area will increase in size by the square for every distance unit. As the surface increases, the intensity becomes dissipated over the larger surface area. This, in turn, reduces the intensity by the inverse of the square, hence we get the equation.
Intensity = 1/ distance²
This principle appears in many areas of science, from Newton’s law of gravitation, to sound waves, electrostatic charges, and all types of radiation. What is interesting about this equation is that it requires a specific unit of one in order to define a scale of measurement. Interestingly, if the distance to the light source is zero, then one divided by zero² will also appear to equal zero. Clearly this cannot be the case. However, in our new theory of Geometric Maths, the concepts of zero² does exist. In fact, it creates the rotation of the number line at 90°, similar to the complex number plane. Similarly, light waves are divided into the E- and B-field that exhibit the same orientation.
The speed of light itself is limited by the electromagnetic resistance of the vacuum, a fact covered in great detail in our article on the subject. This raises two important points, namely, that light is a spherical wave, whose rate of expansion is limited by the speed of light (c). This fact makes it extremely difficult to justify the particle model of light, as the definition of a particle represents a single measurable point in space. This is in opposition to the measurement of light over an area of space, namely the surface of a sphere. Whilst the particle nature of light can be dispensed with, its wavelike nature cannot, and therefore if we are able to resolve the photoelectric effect using the laws of spherical waves, the wave-particle duality theory proposed by quantum physics loses all foundation.
What is the work function?
The work function (W) is recognised as the amount of energy required to dislodge an electron from the orbit of an atom. Each element on the periodic table will exhibit a specific work function value. At room temperature, this varies from around 1.5eV to 5.3eV for the various elements.
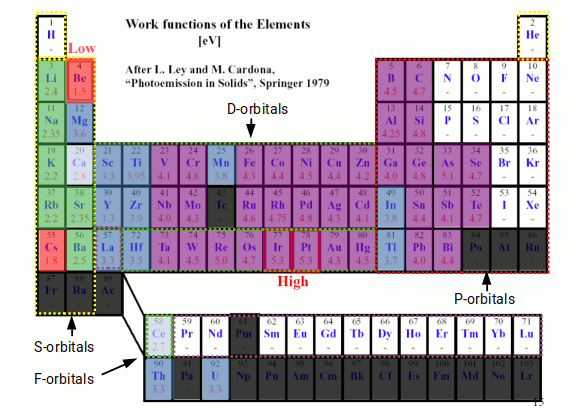
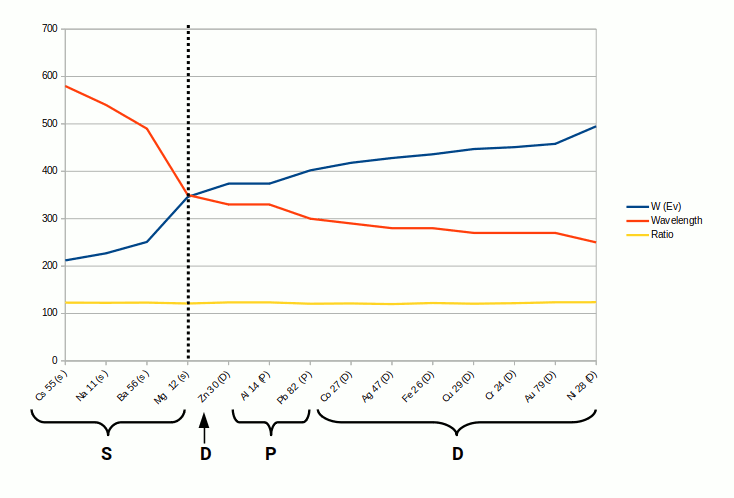
In the image above, we can see the S-orbital elements (left) have a lower work function than other elements on the periodic table. This can be explained as the S-orbital is much more loosely bound than other orbital types, appearing at the outermost shell. Notice, that as these electrons begin to fill the orbitals shells (left to right), the work function generally increases. However, there are certain P-orbitals (right side) that do not exhibit a photoelectric effect. The same can be seen for the majority of F-orbitals (bottom row). Note that element 57 should fall into the F-orbital set, however, due to an anomaly in the Aufbau principle, its electron configuration is formed from D-orbitals instead. Similarly, Element 71 should be in the 3rd D-orbital set.
The elements of the far right are non-reactive noble gases, that do not form bonds with other atoms. These elements are of octahedral structure. The D-orbitals that appear in the mid-section of the table have a cubic orbital arrangement, and, in most part, exhibit a slightly higher work function value. These orbital shapes are explained in great detail in our new Atomic Geometry theory of the atom. If you are not familiar with the periodic table, we also provided a deconstruction of the different orbital types in our new theory of Harmonic Chemistry.
We have coloured each element in the table above to represent the different colour frequencies that initiate the photoelectric effect. Red, green, and blue frequencies are able to initiate the release of electrons within the visible light range. The dark blue and purple colours represent frequencies that are in the violet and ultraviolet spectrum. The work function of a material is directly related to the emission of the photoelectric effect. The higher the work function, the higher the frequency of light needed for the phenomena to be initiated. Furthermore, the work function will vary depending upon the temperature of the material. As the temperature increases, the work function will decrease. This makes sense as in a heated material, atoms have more energy and move more freely, so the electrons are more loosely bound to the nucleus.
Electricity and Light
Most people have heard the term watts (W), amps (I), voltage (V), and resistance (R). However, very few people actually have a correct understanding of the deeper truth behind the relationships of these qualities of electricity. The most common mathematical explanation is volts multiplied by amps equals watts. Whilst this equation is true, it tends to hide the fact that the relationship is also expressed using square numbers. Resistance is multiplied by amps² equals watts, whereas resistance multiplied by watts equals volts². This gives us the correct relationship of amps, resistance and voltage through the squaring of these values.
R x I² = W and W x R = V² therefore I² x R² = V²
This fact is dismissed in almost all electrical equations, as the squared values can be removed to make the calculations simpler. Yet, from the perspective of geometry, the notion of squaring forms a 2D plane. The reason this is important is that the speed of light (c) is limited by the electromagnetic resistance in the vacuum of space. This is determined by two important scientific constants, ε0 (electrical resistance) and υ0 (magnetic resistance). In Dimensionless Science, these hold the values 1/36π and 4π, respectively. However, we find that the constant ε0 can also be defined as 1/6π. When we multiply the squared values together, we get the value 1/9, or energy (E). The speed of light (c), is derived from this value as:
1/(ε0 x υ0) = c² = 1/((1/6π)² x (4π²)) = 3²
Notice, that light was traditionally rounded up to its nearest whole number. More recently, the value for υ0 has been reduced to match the suggested value for the Planck constant. In doing so, the relationship between light and electromagnetism has become even more obscured. The value 4π is actually obtained from the surface of a sphere with a radius of 1 by the equation r² x4π. This realization reinstates the correct geometry of the spherical light wave, that cannot be accounted for by the particle model of light.
Additionally, we find that there is now a direct relationship between the laws of electromagnetism, where the constants ε0 and υ0 are found to match the notion of amps (I) and resistance (R) in the equations of electromagnetism. This means the notion of voltage is the speed of light in the vacuum, which when squared becomes the value for energy as depicted in Einstein’s famous equation E =m x c².
The mathematics of EM waves
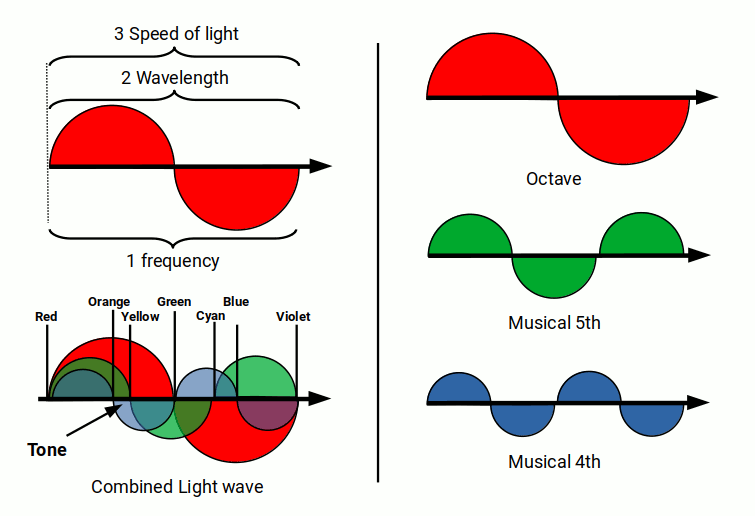
The frequency and wavelength of electromagnetic waves are unified by the speed of light (c). When frequency is multiplied by the wavelength, the result is always c. A wavelength is a distance measurement of space, whereas a frequency is
the number of wavelengths created in a second, a relationship of time. Therefore, a wave always exhibits time:space ratio of 2:1. As the wavelength decreases, the frequency increases, which suggest that the size of each wave is also diminishing, so that the speed of light will remain constant. You can explore the nature of EM wave using this great online calculator.
In the image above, we can see the numbers 1, 2 and 3 represent frequency (time), wavelength (space), and light (Causality). On the left, we find the three types of musical division that generate the 7 notes of a musical scale. The 3:2 ratio is the second type of musical ratio, that determines the musical 5th. Together with the octave, which, when divided in half again, creates the musical 4th, whereby all the notes on a musical scale are completed. It is the distance between the 4th and 5th that creates the musical tone, which appears on the piano keyboard as two white notes separated by a black sharp/flat note. We explore this concept in much greater detail in our explanation of the Ultraviolet Catastrophe.
The bottom left of the image above shows these three ratios combined into a single wave, which produces the 7 colours of light found in a rainbow. When combined, they create monochromatic (white) light. The various shades of colour are formed by the interference of these foundational ratios. More information regarding this nature of light can be found in our new Theory of Geo-optics.
EM waves and electron energy
Every electron in the universe is exactly the same, regardless of where it is found. The electrical charge constant (e) represent a single unit of energy contained in one electron. As the electron is negatively charged, it has an energy value of -1e. The same constant is also applied to the proton, which has a positive charge of +1e. When the combination of these two values is equal to zero, the electron will be in its ‘ground state’, which is the lowest shell in the electron cloud. Each atom in a neutral state will exhibit exactly the same number of protons and electrons, which in turn defines all the elements on the periodic table.
An electromagnetic wave with a wavelength of 200 nm will have a frequency of around 150 petahertz in the near UV spectrum of light. This also equates to an energy of around 1000 zeptojoules, which equates to around 1^18 photons per joule. Each photon therefore has an energy value of 6.24 eV. When this is multiplied by the electrical charge constant, (e), we get the value of 1. The ratio between the energy of a single electron and the photon energy are unified by an EM wavelength of 200 nm, which has a frequency of 1.5 petahertz.
The critical wavelength that triggers the Photoelectric effect. The unification of the electron and photon energy is expressed using DIMENSIONLESS SCIENCE constants below.
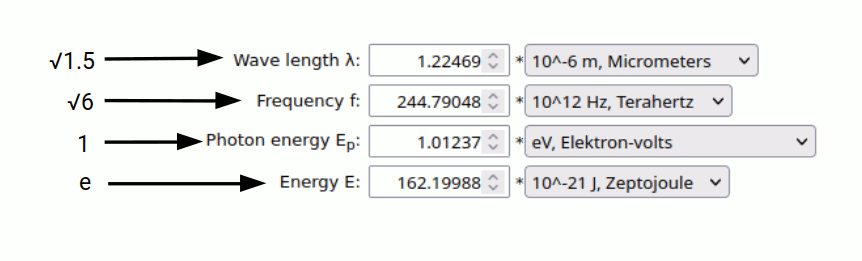
What we can see here is the frequency/wavelength has a 2:3 ratio to the speed of light, which in turn produces a photon energy that, when multiplied by the electrical charge constant (e), produces a single unit of energy (E). Using the values of Dimensionless Science, the photon energy is therefore 2/π. We can set the energy (E) of the EM wave to the electron frequency (160.2), which results in a photon (Ep) energy of around 1. In doing so, we find the wavelength gets reduced to √1.5, producing a frequency of √6.
This established the link between energy (E), photon energy (Ep), to the wavelength (λ) and frequency (f) of electromagnetic waves. What emerges is the ratio between a frequency of 1.5 to an energy unit (E) of 1, which, as f increases to √6, the photon energy now equals 1. The square root of the frequency (1.5) swaps places with the wavelength, which now becomes √1.5, which is also written as √6÷2.
Threshold frequencies
Having explored the relationship between of the speed of light, wavelengths, frequency, photons energy, and the laws of electromagnetism, we are able to examine the nature of the photoelectric effect. We can begin by looking at the minimum frequency for each element, where the photoelectric effect begins, compared to its work function. This is called the ‘threshold frequency’.
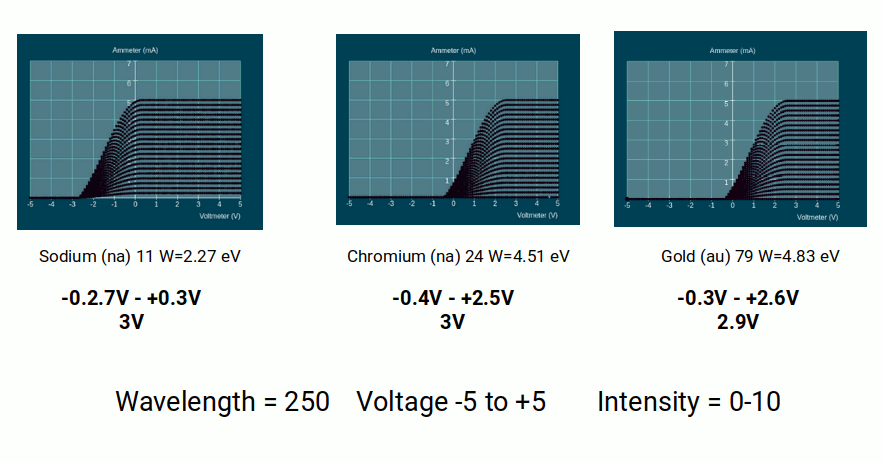
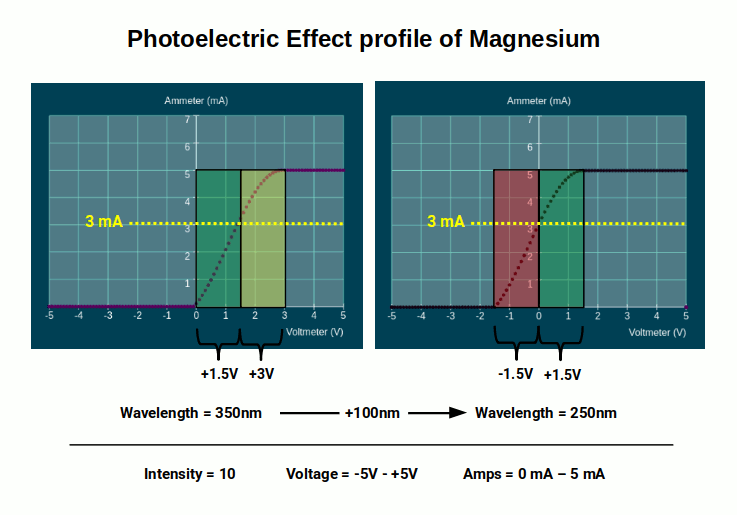
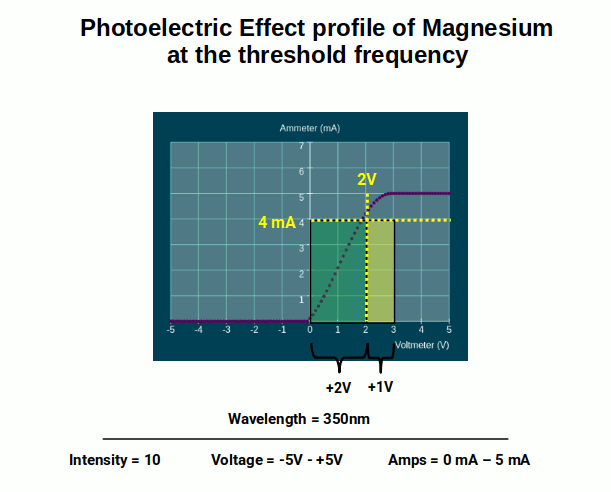
By fixing the intensity of light to 10, we can adjust the voltage range from -5 to +5 for various elements with different work functions. What we find is that the threshold frequencies for each element produce exactly the same curvature, from 0V up to +3V. Increasing the wavelength past the threshold will lower the voltage where the photoelectric effect initiates. We can see that elements with a lower work function will produce photoelectrons further into in the negative voltage range as the wavelength decreases. Yet, at threshold frequency, the curvatures are the same for each element.
Here, we can see sodium, which has a work function of 2.27 eV, begins to exhibit the photoelectric effect once the wavelength of light is increased to 540 nm. Magnesium has a higher work function of 3.46 eVs, therefore the wavelength needs to be reduced further, to around 350 nm, before photoelectrons are produced. Gold with a work function of 4.83 eVs further still. Yet, the curvature from 0V onwards match up exactly for each of these elements, regardless of its work function. In all cases, the curvature levels out at around +3V and 500 mA for the threshold wavelength of each type of atom. We can use the simple mathematical equations that we explored previously to calculate the resistance of the photoelectric effect at the end of the curvature.
I² x R² = V² = 5² x (3/5)² = 3²
The correlation between the voltage and the speed of light (3) is difficult to ignore, especially in recognition of the energy mass equation E=mc². Additionally, we find that the value for resistance, (3/5)², is equal to 0.36. What we recognise is that this value is the same quantity that defines the electrical resistance of the vacuum of space (1/ε0), translated by a power of -100.
(3/5)² = 0.36 = 1/ε0
Furthermore, we find that (3/0.5)π divided by 1.5, (half the speed of light), equals 4π. The frequency of an EM wave of 1.5 produces a single unit of energy, whereas 4π just happens to be the value for the magnetic resistance of the vacuum (υ0), as previously defined in the equation above.
(3/0.5)π ÷1.5 = 4π
What we are beginning to see is that the resistance of the vacuum of space, which limits the speed of light, seems to be related to the photoelectric effect, not the light wave itself, as is commonly believed. This revelation is so profound that it fundamentally changes large portions of scientific thinking. Everything from quantum physics to the production of solar energy is predicated on the photoelectric effect. If the energy is being drawn from the Quantum Foam, this resolves the problem as to why the reaction speed of the emission of photoelectrons cannot be explained by the transfer of energy from the photon to the electron. Light is acting as a catalyst trigger, that causes the energy to be emitted. The picture becomes complete when we find that 6π² ÷ 4π² equals 3/2, or 1.5, the speed of light to wavelength ratio that produces a single unit of energy.
Thus, the true cause for the photoelectric effect is beginning to reveal itself. It is the ratio of the wavelength that causes the emission of photoelectrons. Just as light must overcome the resistance of the background energy in the vacuum in order to propagate through space, so the same can be said of the photoelectric effect.

Book out now!
The Geometric Universe – Beyond space and time
This book unveils the Geometric Theory of the cosmos, from the atom to the galaxy. Filled with intricate illustrations, it offers a concise exploration of the Multidimensional Universe, challenging our understanding of space, time, and existence.
Energy from the Vacuum
The whole universe is filled with a cosmic background radiation (CMB), in the microwave region of the electromagnetic spectrum. Whilst conventionally considered to be a remnant of the big Bang, in our new theory of the 4D Aether we are able to show extensive mathematical evidence that this is not the case. The measurements of spectral radiance shows that the wavelengths and frequencies have a direct correspondence to the speed of light, and electron energy. The CMB can be measured in two ways, through its wavelength or frequency, which produces two sets of results.
Frequency, wavelength, energy, and temperature of the CMB
|
|
Wavelength
|
Frequency
|
Electron Volts
|
Energy
|
Temp
|
|---|---|---|---|---|---|
|
By Wavelength
|
1.87 mm
|
160.23 GHz
|
7.74 x10-6 eV
|
1.24 x10-24 J
|
0.0180 K
|
|
By Frequency
|
1.06 mm
|
282.82 GHz
|
1.169 x10-3 eV
|
187.4 x10-24 J
|
2.73 K
|
When measured in terms of wavelength, the CMB has a frequency of 160.23 which creates a wavelength of 1.87 nm. This wavelength can be generated by dividing 1.5 by 2√3, and then squaring the result, to create the ratio 3/16.
(1.5 ÷ 2√3)² = 0.1875 = 3/16 = CMB wavelength
In the last section, we calculated the resistance of the vacuum as 6π²÷4π² = 1.5, which produces a single unit of energy. This value is now divided by 2√3 and squared to produce the CMB wavelength. The value 3/16 can be represented as the speed of light divided by 4². When 3 is ascribed to the speed of light, this provides a value of 160Ghz for the frequency.
3 ÷(3/16) = 16 = CMB frequency
The second measurement from the CMB is generated by measuring its frequency. This set produces a value roughly equal to √8. This value is obtained by the division of 2√3 by √1.5.
(2√3 ÷ √1.5 ) = 2.828 = √8 = CMB frequency
From this we can determine the wavelength by dividing 3, the speed of light, by the frequency, which gives a value of (3√2)÷4.
3 ÷ (3√2) = (3√2)÷4 = 1.060 = CMB Wavelength
This establishes the geometric nature of the CMB, frequency and wavelength, for both types of measurement. Notice, that in the previous section we saw that a wavelength of 200 nm produces a frequency of 150 Petahertz (1.5), which unifies the energy of the electron and photon into a single unit of energy (E). Whereas, when the values for photon energy (Ep) set to1, the wavelength reduced to √1.5. The values 1.5 and √1.5 for these frequencies and wavelength are reciprocated in the calculation of the spectral radiance of the CMB.
By wavelength: (1.5 ÷ 2√3)² = CMB λ and 1.5 EM f = 1 Energy (E)
By Frequency (2√3 ÷ √1.5 ) CMB f and √1.5 EM λ = 1 Photon energy (Ep)
As the multiplication of the wavelength and frequency of an EM wave, always result in the speed of light, we can recognise that λ and f are inverted. The wavelength measurement is squared, whereas the frequency measurement employs √1.5. Both express the number 1.5 and 2√3, which is unified to photon energy (Ep) and energy (E). Could it be this correlation is just a coincidence? Much effort has been put into making us think of the CMB as existing in the void of space. However, the truth is it is also found in the space that directly surround us. Everything in the universe is emersed in the energy, including all atoms. For this reason, we can postulate that it could be intimately related to the photoelectric effect, which is not produced by light, but is in fact extracted from the vacuum energy of space.
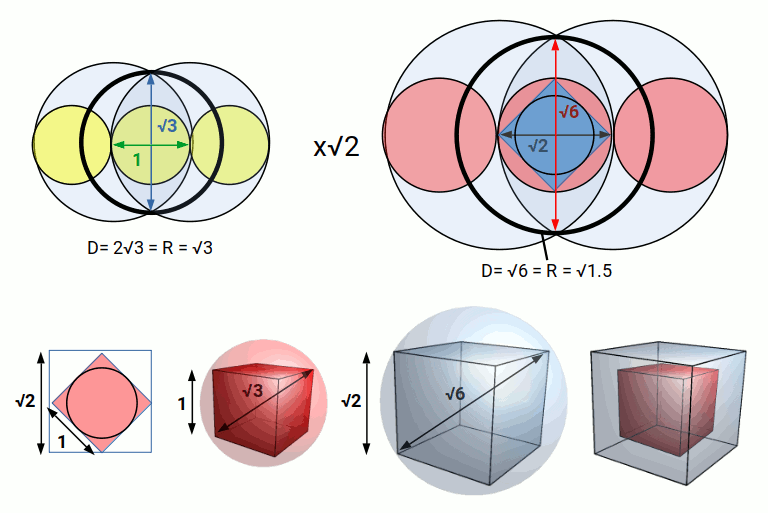
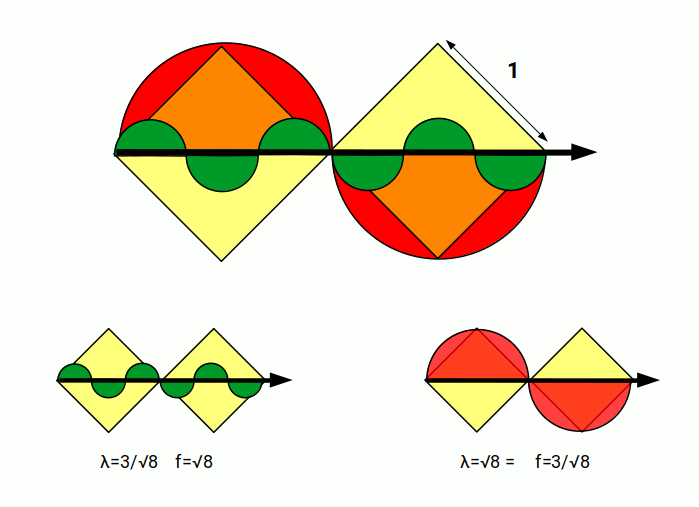
The reasons for this becomes clearer once we adopt a geometric view of this relationship. A square with a side length of 1 will measure √2 across its diagonal. A second square rotated at 45° can perfectly encompass this, with a side length of √2, a ratio of 1:√2. However, in 3D, a Cube with a side-length 1 will measure √3 across its furthest diagonal. Whilst, a Cube with side length √2 measures √6, which when halved becomes √1.5. When viewed in 2D, we find these same relationships are expressed as the distance between the nodes of two interlocking circles, produced at the scales of 1:√2.
The top left image above shows three circles (diameter 1) that create a line (distance 3), representative of the speed of light. The thicker mid-circle has a diameter of 2√3. When this image is scaled by a factor of √2, the mid-circle now exhibits a diameter of √6, and radius of √1.5. Below we show the same ratio expressed in a 3D cubic space. The image on the left shows a Cube side-length 1, nesting inside another Cube side length √2, forming a particular type of Hypercube (4D tesseract). Therefore, the CMB frequency of √8, or (2√3 ÷ √1.5), is just a transformation of scale from 1 to √2.
With this foundational comprehension of the geometry of space, we can now start to resolve the unification of the CMB to the energy (E), and photon energy of the EM waves. As explored previously, an EM wave with wavelength 200 nm creates an energy (E) value of 1, whereas when this is reduced to √1.5, we find the value of the photon (Ep), 1.
On the right-hand side of the image above, we can see the wavelengths of 1.5 and 2, that divided the line, which represents the speed of light, into 4 and 3 sections, creating the musical 4th and 5th, respectively. On the image to the left, the musical 4th is scaled down by a factor of √2 to produce a wavelength that expresses the value of a single photon. Notice, that the first two frequency lengths now fall short of the value for the speed of light. The 5th circle does not complete. √6 has a value of 2.449…, so the 5th circle only covers the distance of √6-2. Amazingly, when this remainder is multiplied by π/2 to find the half circumference of this additional part of the wave, we find the result is extremely close to ½√2.
√6-2 x ½π ≈ ½√2
The frequencies of the CMB and its relationship to the electromagnetic resistance of the vacuum become much easier to understand when represented in a geometric format. Previously, we saw how musical ratios can be expressed as a string divided into 2 and 3 to create the octave, the musical 4th, and 5th.
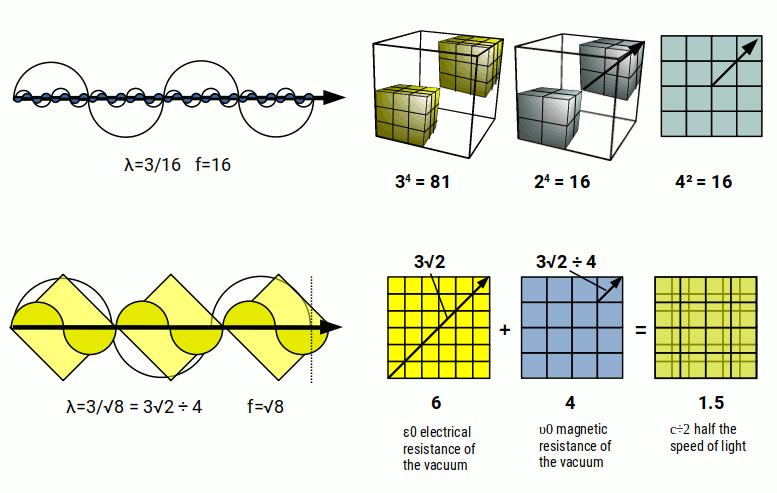
The same principle applies to the CMB. A frequency of 160 produces a waveform that divided the length of a line (speed of light 3) into 16 wavelengths. This is therefore derived by dividing the line of 3 to produce 16 small wavelengths. The second value of the CMB has a frequency of √8, which is again divided into 3 to produce the wavelength. √8 is the diagonal of a square side-length 2.
Therefore, a square of 4 which has 16 units will have a distance from its centre through it diagonal of √8. These ratios can be achieved by superimposing a square, side-length 6 over a square side-length 4. In simple terms, the values 6 and 4 equate to μ0, and √1/ε0, (excluding π), that create the electromagnetic resistance in the vacuum of space. Notice, that the speed of light (3) is the distance of half the square of 6, as science tends to only measure the expansion of the spherical wave based on its radius.
The image above (left) shows the two types of EM wave that form the CMB, which are derived from the division of a line into 2 (blue) and three (yellow). On the left, we find the 2D correlation to the squares that form these wavelengths. As 16 is also 24 we can also represent these quantities as two types of Hypercube. In Geometric Maths, the notion of powers is directly related to dimensions of space.
Interestingly, all matter in the universe is composed of only 81 stable atoms formed from a maximum up to 16 electron pairs in a single shell, which is derived from 34 and 24 repectivly. This visual representation of the CMB unifies its qualities with the resistance of the vacuum, which limits the speed of light (causality), through a hyper-cubic construct, which ‘orientates’ the atoms and electrons of the whole universe into a unified moment of time.
Harmony and Resonance
The concept of resonance shows us that a wave at a particular frequency can affect another wave that exists at a different scale. This can be demonstrated with any stringed instrument, such as a guitar. By placing a finger on the second fret of the second string, we create the interval of the musical 5th. When the 1st string is plucked, the second string will also vibrate. If we move a finger onto the 1st fret, a semitone below the 5th, and try to repeat the experiment, then the effect ceases to be apparent. This is most apparent with the octave, and musical 4th.
The reason for this is simple. A wave that is combined with another of a different frequency creates an increase in the overall amplitude, where the peaks fall into the same place. This is called constructive interference. As a wavelength of the octave combines at the halfway point, it increases the overall amplitude of the wave. The concept of resonance has a vast range of application, including acoustics, electrics, EM waves and atomic physics.
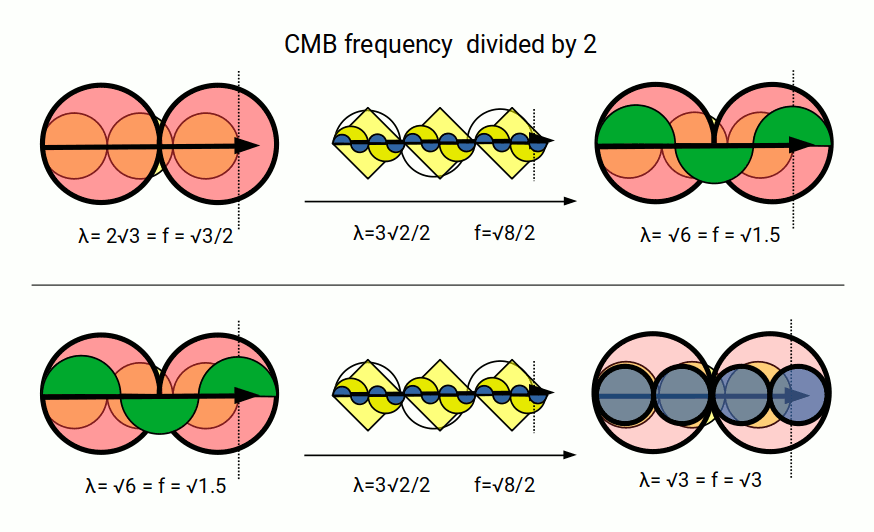
The photoelectric effect exhibits a similar correlation to the frequencies of the cosmic microwave background, which has a frequency of √8. The EM wave that initiates the flow of photoelectrons with magnesium has a frequency of √3/2. When this is divided by a frequency of √8/2, (the half that of the CMB), the result produces a wave with a frequency of √1.5. Dividing this new wave again by the same CMB frequency, reduces it to √3. Now, the frequency and wavelength fall into equilibrium, both exhibiting a value of √3.
Scaler waves
Thus far, we have provided a substantial amount of evidence that supports the theory that the photoelectric effect is driven by the energy extracted from the cosmic microwave background; a 4D Aether. We have examined Magnesium, as its work function appears to be in ratio to its threshold frequency, which, when the two are multiplied together and then squared, results in half the speed of light. We also revealed that c/2 is unified to the wavelength of the CMB, as 1.874 roughly equates to √1.5÷10³.
The reasons for this are explained by the structure of an EM wave and its relationship to the musical octave and 5th, or more simply, the division of a line into 2 and 3 equal parts. We saw previously that when two circles overlap equally, the distance between their intersection is √3. This forms the basis for a new set of waves, from which a 2√3 wavelength is produced. Again, this becomes much easier to comprehend once expressed in terms of a geometric image.
This new EM wave is scaled by a factor of √3, which means its wavelength is 2√3, or 346 nm, which equates to the work function of magnesium. When we examine the qualities of this EM wave, we find the number of photons per joule is also about √3. Furthermore, as the value for 2√3 is actually equal to √12, when squared, the result (12), which is the atomic number of magnesium, i.e. the number of electrons, protons and neutrons that constitute the atom. When the number 12 is squared again, the result, 144 that is only 6 nm short of the 150, which is the result of the work function equation. As an interesting side note, 144 also happens to appear in the Fibonacci Series, and in our observation, is the limiting factor for the growth formations of the plant kingdom, often depicted in the sunflower.
In order to make greater sense of the reasons behind this relationship, we will examine how a shift in wavelength changes the profile of the photoelectric effect. As noted previously, from a range of 0V to +3V, we find there is an increase in current, from 0 mA to +5 mA. By reducing the wavelength a from 350 nm to 250 nm, we notice that the curvature shifts to the left. Now, instead of beginning at 0 volts, the curvature now starts at -1.5V up to +1.5V. We have reduced the wavelength by 100 nm, which shifts the curvature by a half to the left.
A wavelength of 2√3 (346 nm) when divided by √2, becomes √6, or 244.9 nm, which is close to the 250 nm mark, that shifts the photoelectric curvature to the left by a half. If we again scale this wave by √2, the curvature moves to the left by yet another half, and we end up with a wavelength and frequency that both equal √3. Therefore, the EM wave is being scaled by a factor of √2, which, after two iterations, reduces the size of the wavelength by a half and shifts the photoelectric curvature by -3V to the left.
Here, we can see that the EM wave is scaled to √3, compared to the speed of light (3). As the wave diminishes at the rate of √2, the middle wave divides into a single wavelength into 3, and then 4. Exactly the same principle is also expressed in the construct of musical scales, which creates the octave, 5th, and 4th. This structure can be applied to a wave in unity with the distance of the speed of light, which, in this case, is scaled by a factor of √3. We can call this a scaler wave.
Below, we have included the Dimensionless Science values, which make calculating these waveforms much simpler, as the speed of light is rounded up to 300,000 km per second. Notice, that at the midpoint, the photon energy equates to a value of 5, which as it has a ratio of 2/π greater than energy, means E approaches the number 0.8 (or 0.785). The value 0.8 equates to a fraction of 4/5, which differs from the value of 0.5π/2 by only 1.0185.
Additionally, the number of photons per joule is 1.25 which is the fraction 5/4, the reciprocal value of energy. What we are beginning to see is that there is a ‘sweet spot’ at the halfway point that unifies π with the numbers 4, 5, and 8. Geometrically, the 5-pointed star creates the Golden ratio, whereas the 8-sided octagon produces the Silver ratio. The former is formed by √1.25±1, whereas the latter √2±1.
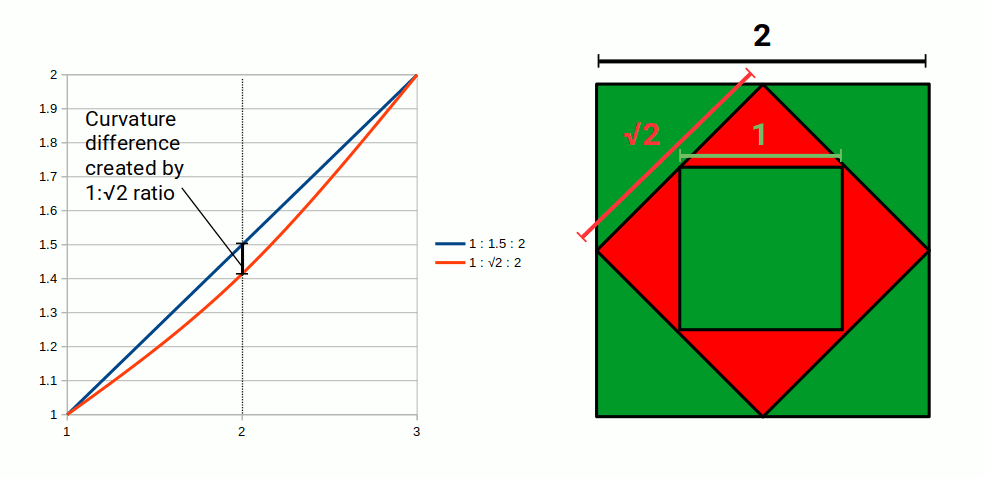
The wavelength of the CMB exhibits two values. The first, of 1.87 mm, is very close to √(2√3), differing by only 1.004. The second wavelength is 3/√8, that when multiplied by √2 equals 1.5. What we can see is that in both cases, EM waves do not scale through the process of halving, but through the ratio of √2, and √3, which are musical and geometric ratios, relating to the square and the Cube respectively. This suggest that electromagnetism is ordered by the principles of geometry, and music, as a function of scaler waves.
The image above shows the slight difference between a linear division of a square through its diagonal, and the division of a line through the ratio of √2, which is found as the scaling factor of the nested squares to the right.
4D Waves
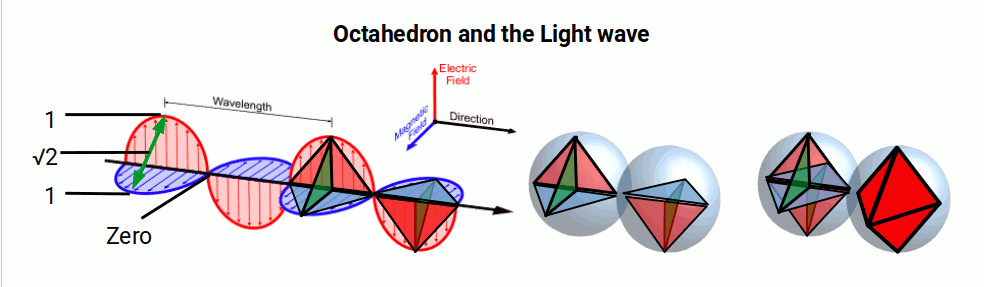
The reason for the appearance of the ratio 1:√2 has to do with the nature of electromagnetic wave themselves. Each wavelength comprises an electro and magnetic component, an E and B field, that are off set at 90° to each other. As these expand in unity to a maximum distance from each other, the distance between each grows at a ratio of 1:√2. From the perspective of the 4D Aether, this forms the shape of an Octahedron. We call this the Octahedral Light.
It is this nature of EM waves that causes the continuous expansion and contraction of the fabric of the background Aether, that transmits the energy through the vacuum of space. As the EM wave progresses through space, each component wave expands and contracts at 90° directions over the course of a single wavelength, before repeating the cycle. This produces the geometry of a quarter section of Octahedron.
Two Octahedral complete the wave’s cycle. Similar to an electron with half spin, whereby a 720° rotation equates to a single rotation of 360° in 3D space, the octahedron is also required to rotate twice to fulfil one rotation in 3D. This is called 4D rotation, the concept of which is applied to all particle spin found in standard quantum mechanical theory. In our 4D geometric model of the universe, this is attributed to the rotation of a 4D object in 3D space, which includes all virtual partials, including EM waves.
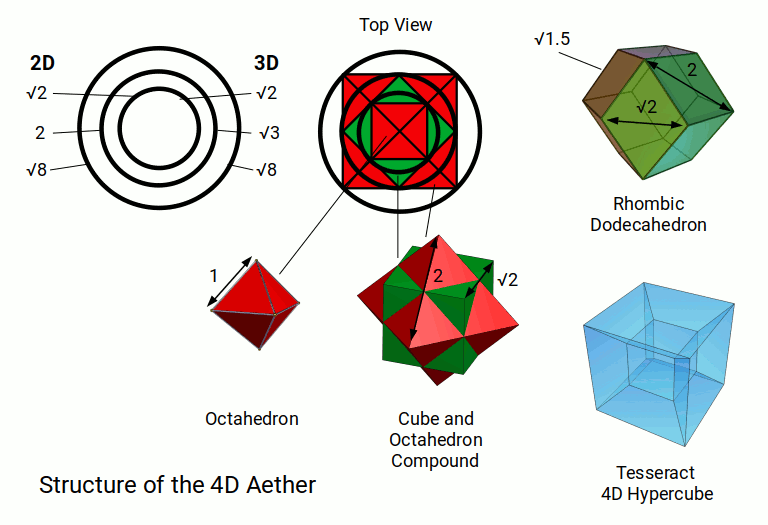
If we complete the larger picture, 6 Octahedra combine to form another Octahedron twice the size. This structure can fill 3D space leaving 8 tetrahedral gaps into which a small Cube can form, This creates a compounded polyhedron, comprised of a Cube and Octahedron. By connecting the corners of the Cube and Octahedron compound, the Rhombic-Dodecahedron is formed, which is the 3D template for the 4D Hypercube, or Tesseract. Notice, that if an octahedral unit has a side length of 1, the Rhombic-Dodecahedron has a side length of √1.5. The Tesseract is composed of two Cubes, often portrayed in 3D as one Cube nested inside of one another.
The ratios shown in the top left shows the diameter of the circle in 2D or the Sphere in 3D. Notice that in 3D, the mid-ratio changes from √2 to √3 due to the protruding corners of the Cube. As the light waves expand and contract, the universe is set into constant motion through the expression 1:√2 present in all EM waveforms. This means, that this 4D structure is also present at the scale of 1 and √2, which produces the quantised state of the wave function.
This explains why quantum mechanics must ‘normalise’ their equation using √2, which is a key point in explaining Bell’s theorem. When two light polarisers are placed at 90° to each other, blocking out the EM wave, and a third is placed in between at 45°, the light suddenly becomes visible again. The online experiment clearly shows that the result of √8 predicted by quantum physics. This can be explained by the geometric structure of light, rather than proposed theories which result in faster than light communication.
This ratio of 1:√2 is easily defined geometrically as a set of squares nested inside each other at a 45° angle. We call this the √2 fractal. Notice, that the √2 fractal creates the ratio of the musical 5th and octave. This is translated into 3D space in the compound of the Cube and Octahedron, which defines the Rhombic-Dodecahedron and creates the template for hyper-cubic space.
Just like a perfect geometric jigsaw, this template is found within our new theory of Atomic Geometry and is key to Geo-Quantum Mechanics, which is able to produce a model of all the stable elements that is up to 500% more accurate than the Bohr and Van Der Waal model in terms of atomic radii. We can create two versions of this template, one based on a cube side length 1, and another based on a cube side length √2. This form a hypercubic model when the 2 cubes are superimposed over the same centre.
By examining the ratios, we can see that we have defined all the components needed to explain the nature of the CMB, and the EM waves that unify the photon energy. What we can see is, that it is √1.5 that unifies the two scales. As the light wave is considered octahedral in nature, it passes through this compound, forming the sphere √1.5 that is found to surround the Rhombic-Dodecahedron in the first instance, and the Cube in the second. This unified ratio enables the rotation of the two Hypercubes to act in unity at the scales of 1:√2, which is found in the nature of electromagnetic waves. This outlines the theory of the 4D Aether, which is the nature of space that ‘quantises’ the light wave, acting as a medium to transport EM waves through the vacuum of space, limited to the speed of light (3).
Work function-wavelength equation
The relationship between the wavelength of light and the work function of different materials also exhibits a specific ratio. By simply multiplying the work function by the wavelength of the threshold frequency, the result can be squared to produce a value roughly equal to half the speed of light (1.5).
The graph above shows this relationship. Note, that the ratio (yellow) is divided by 1000 in order to provide greater clarity of the relationships between the work function (blue) and the wavelength (red). The fact that the yellow line lies on the horizontal plane, shows that for the 14 elements listed, the correlation between wavelength and work function holds true. This provides a new equation that now relates the speed of light to the work function of various materials, and the threshold frequency that initiates the photoelectric effect.
(Wλ)² = c/2
This means that the work function multiplied by the threshold wavelength is equal to the speed of light divided by 2. This also means that the wavelength multiplied by the work function produces the value √150, the point where the point where the EM wave unifies with a single unit of photon energy.
Notice, that we have achieved this unification without having to resort to the mysterious Planck constant. Instead, the result is calculated through simple ratio. The implications are that, whilst the Planck constant can provide a theoretical solution for the photoelectric effect, there is an alternative. As the concept of a photon particle of light and wave-particle duality are predicated on the solution for the threshold frequency of the photoelectric effect, to produce an equation that offers a clear correspondence casts a large shadow of doubt on the theory.
Through this equation, we realise that when the work function is multiplied by the threshold wavelength, the result is √1.5, which is the value that unifies the hyper-cubic scales of reality. The value of √1.5 also forms the side-length of the Rhombic-Dodecahedron at the √2 scale. Additionally, this wavelength produces a photon energy of 1 in an EM wave.
The conclusion is that the Planck constant, which defines the energy of a photon, is about ratio, not quantity. This is confirmed in our examination of the reason why the laws of classical electromagnetism failed to predict the experimental curvatures of black body radiation. It turns out that Rayleigh and Jeans employed the harmonic series in their calculation rather than harmonic ratio.
We are also able to link the work function-wavelength equation to the wavelength for the CMB of 1.87 mm. When raised to the power of 4, the result is very close to √1.5.
1.874 = 1.235÷10³ ≈ (√1.5 ÷10³)
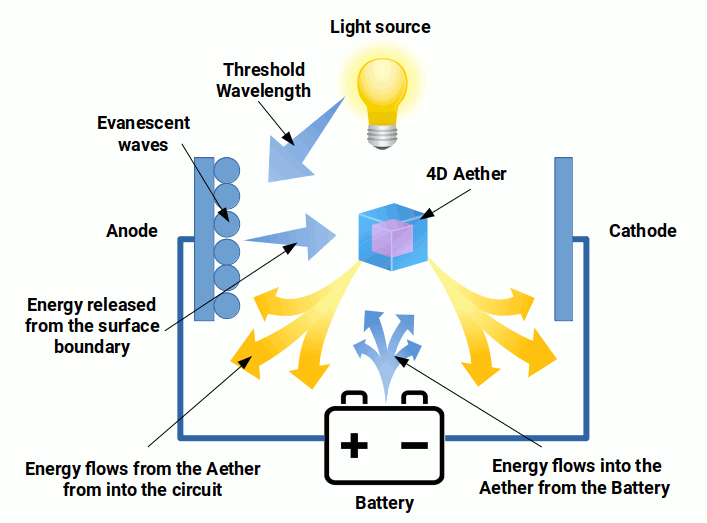
Therefore, the solution to the photoelectric effect can be resolved as the interaction of the 4D Aether at the surface boundary of the material. At the atomic scales, we find that all solid matter is bounded by evanescent waves. When EM waves of a particular scale hits this surface, it causes a disturbance of this field, drawing energy from the vacuum. We suggest that a photoelectron is the ejection of energy from the simulation of this surface boundary. This is called extracting energy from the vacuum of space.
If our theory of 4D Aether should be found to be true, then it turns out that we have already discovered the secret of extracting this potentially infinite energy source. All that remains is for us to improve the methodologies by which this energy is obtained.

Inspiring Events
Whether in person or online, our team is bringing conscious connection and inspiring ideas to beautiful locations worldwide. From scientific talks to creative workshops, learn how to elevate your intellect to bring positive change through geometric thinking.

Talks & Webinars
Explore the intricacies of our groundbreaking discoveries and join the discussion as we are solving modern scientific enigmas to unveil deep philosophical insights.

Retreats & Trainings
Embark on a transformative journey where ancient wisdom seamlessly intertwines with cutting-edge science, all rooted in the mesmerizing tapestry of the Geometric Universe.

Workshops & Courses
Dive into hands-on learning with our creative Sacred Geometry workshops and courses! Unlock the mysteries of the universe in a fun and accessible way suitable for all ages.
The geometry of Magnesium
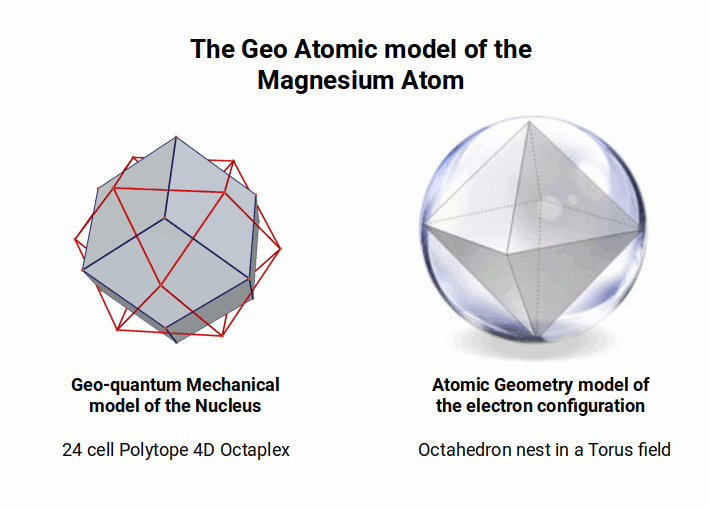
When we examine the work function relationships to the wavelength of the threshold frequency in the previous table, we notice that the two lines intersect for the element of magnesium. This atom has an atomic number of 12, which means it has 12 protons, and 12 electrons. Its most stable isotope also contains 12 neutrons. According to our new theory of Atomic Geometry, a magnesium atom exhibits the structure of an Octahedron, (complete set of P-orbitals), surrounded by a 4D Torus field, (pair of S-orbital electrons).
From the examination of the work function of different elements, we can understand that these types of elements tend to exhibit a lower work function than others on the periodic table. This is because the frequencies of the photoelectric effect tend only to effect the outmost electron shell. In our theory of Geo-Nuclear physics, the 12 neutrons and protons of magnesium forms an atomic nucleus that has the qualities of a 24-cell 4D-polytope, the Octaplex. This can be represented in 3D space as a Cuboctahedron and Rhombic-Dodecahedron dual. The magnesium atom also happens to have a radius of 150 picometres, and so a diameter of 300pm. Therefore, we find a correlation between the size of magnesium, and the Wf² equation and the speed of light.
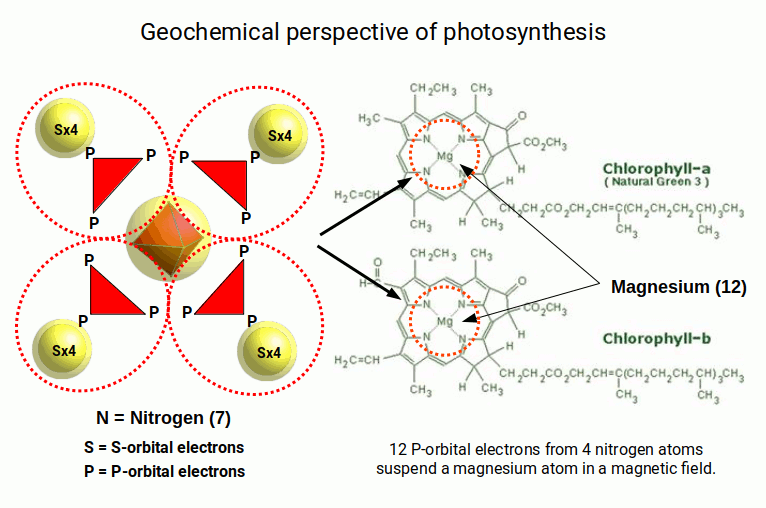
Magnesium has a radius of 1.5Å and therefore a surface area of 9π, the value for energy (E) multiplied by π. This might explain why magnesium is the atom is central to the process of photosynthesis, the mechanism by which plants convert sunlight into energy. Within the chlorophyll molecule that is responsible for this process, we find a single magnesium atom suspended in a magnetic field by 4 nitrogen (7) atoms. When struck by sunlight, this causes the magnesium atom to vibrate. The energy is subsequently used to ‘split’ a water molecule.
What we have suggested so far completely changes the perspective of the photochemical process inherent throughout nature. Plants are not converting energy from sunlight. Instead, they are using the frequencies of light to vibrate the magnesium atom, which is extracting the energy from the ‘vacuum’ of space. This is then used to split a water molecule, the energy of which powers life.
This model begins to explain why the structure of different compounds and molecules can have a dramatic effect on the work function of a material. If the energy is being drawn for the background Aether, then the geometry of the material starts to play a more important role.
This also explains the nature of Photoelectric Fatigue. Over time, the number of photoelectrons produced reduces. It has been shown that this is directly related to the material itself, which, over time, produces a thin oxidation layer. This plays a key role in the development of semi-conductive materials, The conclusion is that it may well be the disturbance of the evanescent waves at the surface boundary of the material that causes this effect. If this is the case, then the process that causes a metal to rust, or a battery to lose its charge, now takes on a completely different perspective. As new research is now starting to bring to question the validity of the traditional view of photoelectric effect, we find that this new 4D Aetheric view satisfies many of the strange anomalies that are presently being uncovered.
Squaring the circle
Now that we have explained the nature of scaling through √2, we can see that the halfway point is slightly under the value of 1.5. Whilst the magnesium atom has a work function of 2√3, the wavelength that triggers the photoelectric effect seems slightly higher, at around 349 nm. This about 3.5 nm greater and produces a frequency of around 857 THz. When squared, we notice that the result is equal to roughly √3-1. When this is scaled by a factor of √2, the result is 121nm (interestingly close to 11² or 121). Furthermore, when we divide this result by 2√3, we get 349, which is the original wavelength of the EM wave, scaled by a factor of 10.
√f x √2 = 1.21 and 1.21÷2√3 = 0.349
EM wave f =√3-1 λ =3.49
Mg work function = 2√3
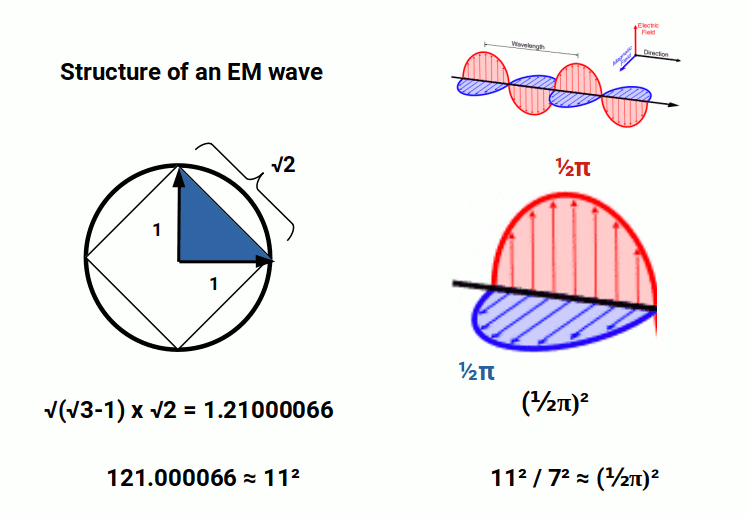
The value √3-1 also relates to the heat/frequency relationship of the cosmic microwave background. Measure by frequency, the CMB exhibits a temperature of 2.73 (√3+1), whereas its frequency of √8 (282GHz) These can be placed on a right angles triangle with √3-1 at the base, which is the frequency that triggers the photoelectric effect for Magnesium.
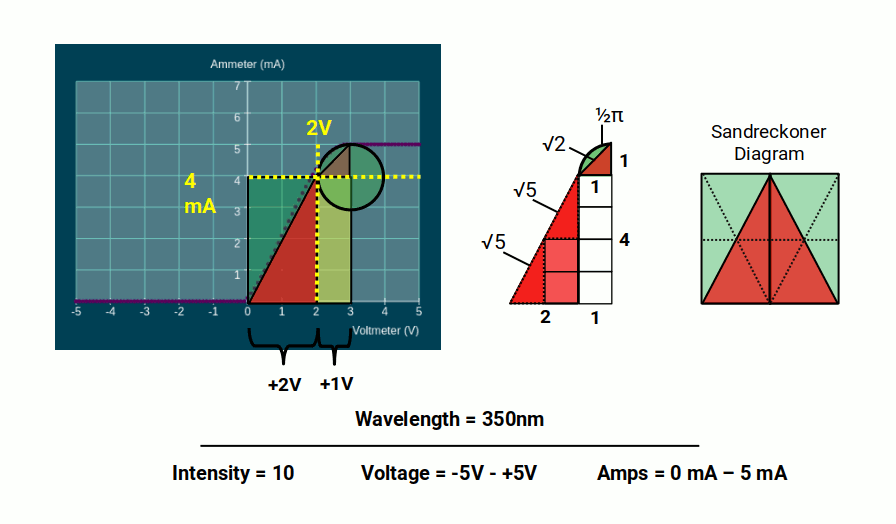
There is a small geometric secret that begins to explain why this should be so. When the frequency of 0.856, or (√(√3-1)), is multiplied by √2, the result is 1.21000066. The value 121 is the result of 11². When divided by 7², the value approximates ½π². As EM waves are formed of an E and B field offset at 90° to each other, the maximum distance between the E and B field becomes √2. This explains the multiplication of the threshold frequency by a factor √2. As π is the relationship between a circle’s diameter and its circumference, ½π equates to the distance of a half circle. Finally, the value is squared when these two waves are multiplied together. The result is that the circumference of a single half-wave is ½π², which can be approximated by 11²÷7².
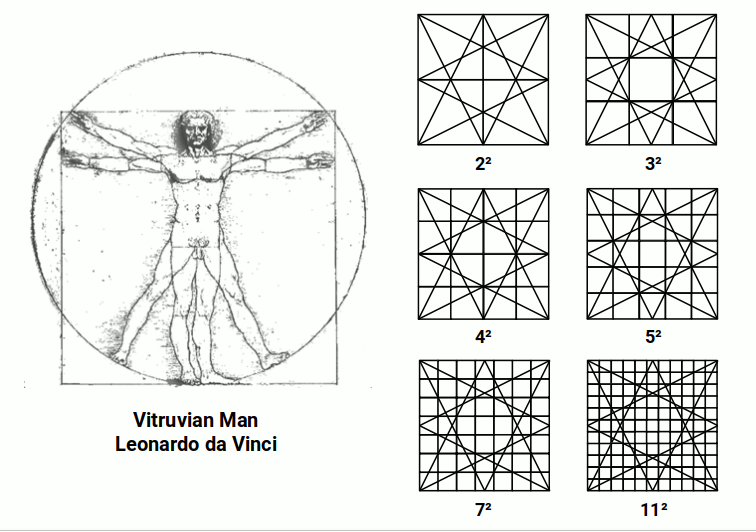
We have identified the appearance of 11², which has a value of 12, just over 120, and just under √1.5, which has a value of around 1.22. So it seems there is a ‘sweet spot’ as we approach this value where the photoelectric effect beings. Previously, we noted that the wavelength of √6, and a frequency of √1.5, with a photon energy of around 5. As this central wavelength increases ever so slightly, the photon energy decreases to 4.9 which is 0.7². Therefore, the relationship between a frequency of 12.1 and a photon energy of 4.9 appears at around the same point. In simple terms, the value 7²=49 is derived from the photon energy, and the value 121=11² from the frequency of the wave. The squaring of the surface area of the circle is derived from this ratio. This is expressed geometrically in something called the Sandreckoner diagram, and is also the process used in da Vinci’s famous drawing, the Vitruvian Man
Here, we can see that the squaring of the circle is produced through a particular geometric function, where 11² is used in conjunction with 7² to form the π ratio. In Leonardo’s famous drawing, the circle has roughly the same circumference as the perimeter of the square. A point where the straight line meets the curve. Similarly, EM waves exhibit a circular geometry. The electrical permittivity ε0 and the magnetic permeability μ0 do not depend upon direction, polarization, or field strength, therefore free space (vacuum) is isotopic, i.e. flat. Thus, we can begin to see that the extraction of energy from the vacuum, requires a transformation between the circle and the square.
Whilst this might seem like a rather strange coincidence, there is actually a logical explanation. The photoelectric effect operates at the boundary between matter and energy. When the wave form falls into resonance with the square, energy is extracted from the vacuum of space.
It is quite amazing to think that in traditional geometric terms, the square was considered to represent the world of matter, and the circle the world of the spirit, the heavens, or in modern terms, energy, and the conflux between the two, a divine mystery. In light of this new theory, this might still offer the solution, as the point where matter is transformed into energy.
Cubing the surface of a Sphere
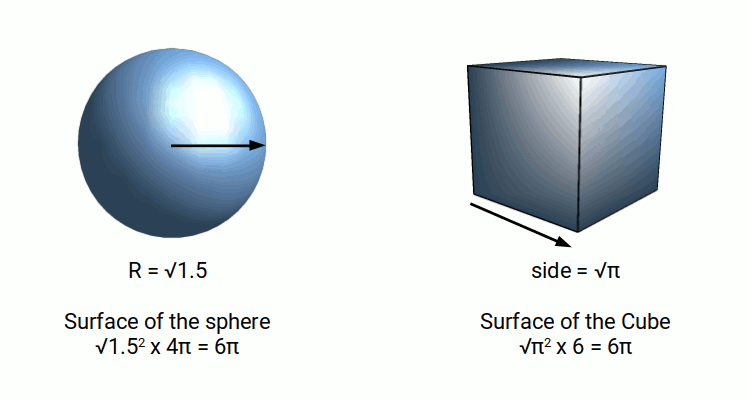
The area of a square is produced by raising the side length by a power of 2. For example, 2²=4 is the surface area of the square with a side length of 2. The same applies to any other number that is squared. Therefore, in order to produce a square with a surface area of π, we need to find a side length of √π.
√π² = π
A cube has six sides. Therefore, the surface area of the cube is found by multiplying the area of a single side by 6. For a cube whose side has a surface area of π, the total surface therefore becomes 6π.
√π² ×6 = 6π
The surface area of the sphere is found by the equation r²×4π, where r is the radius of the sphere. If we set the radius to √1.5, the surface area of the sphere will be 6π. What we see is that the critical wavelength that initiates the photoelectric effect is the radius of the sphere that produces the same surface area as a cube with a side length of √π.
Previously, we explored the concept of the squaring of the circle. When this concept is translated into 3D space, we find that the sphere’s radius becomes √1.5. The point where the surface areas of the sphere and the cube unify. A sphere with radius √1.5 produce by light thus falls into harmonic resonance with a cube produced by the lattice structures of matter. When brought into resonance the energy from the vacuum begins to be extracted, initiating the photoelectric effect.
The CMB and the fine structure constant
One of the most fundamental constants in science is the fine structure, denoted by the Greek letter α (alpha). This is a dimensionless constant that denotes the coupling of the electron to the atomic structure. Traditionally it has been given the value of 1/137, yet the reason for its precise value is a mystery in traditional scientific thinking.
The critical wavelength of the photoelectric effect can also be calculated using this constant, when divided by the frequency of the Cosmic Microwave background, √8. The equation can be formulated so that the constant can be divided by the work function (W) to produce the threshold wavelength (λ), or inversely, divided by the threshold wavelength to produce the value of the workfunction in eVs.
(√8 ÷ (α/π)) ÷ W = λ
(√8 ÷ (α/π)) ÷ λ = W
CMB frequency = 2.8282 = √8
Notice that α needs to be divided by π to create the correct result. In Dimensionless Science, the value for α is found as 4π÷√3. The division by π transforms this into the simple fraction 4÷√3. When √8 is divided by 4/√3, the result is √6/2, or √1.5
√8 ÷ 4/√3 = √6 ÷ 2 = √1.5
This illustrates a clear relationship between the Cosmic Microwave Background, and the coupling of the electron to the atom. This suggests that the energy produced in the circuit does not come from the EM wave. Moreover, it is being extracted from the vacuum. The relationship between the work function and wavelength are both defined by the ratio of the fine structure constant and the uniform frequency of the CMB this fills the entire universe.
Gravity and Magnitude
Many of the calculation we have discussed have been simplified in order to make the comprehension of the wave solution to the photoelectric effect easier to comprehend for the lay person. The calculations are presented in terms of simple geometric ratio, based on the constants of Dimensionless Science. This produces accurate results without consideration of magnitude. The advantage of this, is that we can focus on the geometric relationship between various constants, and show simple examples of the concept.
The formula (Wλ)² = c/2 actually produces a result that is out by a factor of 100. This is acceptable if we consider the speed of light over a different period of time. Normally, the speed of light is defined in meters per second. Yet these values are a human definition. If we adjust the measurement to kilometres per second, the value for the speed of light decreases by 1000. The same can be said if we decrease the value of a second to a millisecond, the value for light increases by a factor of 1000. It all depends on the time frame and scale of distance we are using to define the speed of light.
However, for a more precise definition, using the accepted magnitudes of c, if we include the gravitation constant into the equation. This is because when c/2 is multiplied by the gravitational constant (G), the result unifies almost exactly to 1/100.
(c÷2) × G = 0.0100044
This means we can rearrange the equation to that the results produced will create the correct order of magnitude for the relationship between the work function and the threshold wavelength.
(√(c² × G) ÷2) / W = λ
(√(c² × G) ÷2) / λ = W
This expression of the wave solution to the photo electric effect is much more satisfying, as we can define both the work function, or the threshold frequency with exactly the same equation. However, this also points to a new correlation between gravity and the speed of light. This is much more clearly express in terms of dimensionless science constant. The gravitational force is defined as 2/3, whereas the speed of light has a dimensionless value of 3. The constants when expressed in these terms are exactly equal to the results produced by the fine structure solution.
(√(3² × 2/3) ÷2) = √8 ÷ ((4π √3)/π)
Gravitational solution = Fine Structure solution
Furthermore, the gravitation solution provides a direct correlation to newton law of gravitation. The gravitational constant is multiplied be the mass of an object, which is divided by the square of the distance between the two objects.
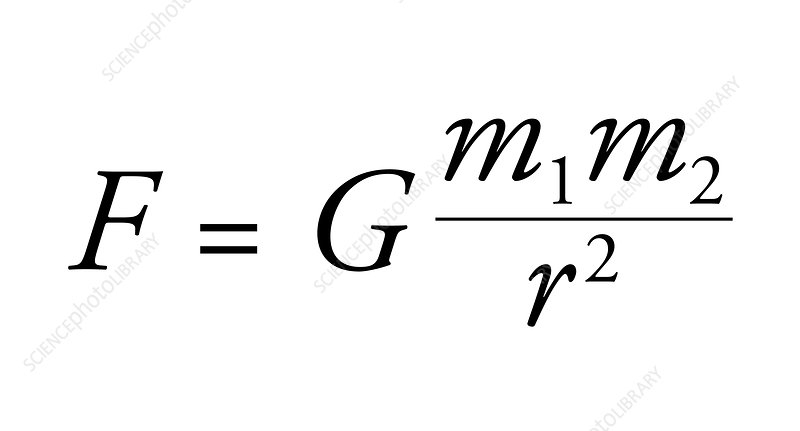
In this case the distance (r²) becomes √2. This is understandable, the √2 is the ratio through which the light waves reduce in scale to create the musical 5th. The equation is expressed as:
√G × (((√c × √c) /2) ÷√2²)
This is almost exactly the same as the law of universal gravitation, with one key difference. The gravitational constant is expressed in its square root form. It is interesting to note that at the cutting edge of string theory, the unification of the electron magnetic field and the force of gravity has recently been unified through the √G.
√G is also found in the expression of the escape velocity, through the equation, V esc = √(2GM)/r, where V is velocity, M is mass, and r is the distance from the centre. If we substitute M with the c², i.e. energy, then divide the result by the CMB wavelength we produce the same result.
Photoelectric effect =√(3² × 2/3) ÷2
CMB relationship = √(2 × 2/3 × 3²) ÷√8
Escape Velocity = √(2G × c²) ÷ CMB f
What this begins to express it the notion that the energy is already inherent within the atomic structure of the atom. When the threshold wavelength is reached, energy is released, which causes the current to flow into the 4D Aether. The energy is then absorbed back into the circuit. Electricity does not flow through the wires, it is conducted through the Aetheric fields and is absorbed uniformly throughout the connected circuit. This concept is discussed in greater detail in our post on Quantum Foam.
Overview of the conceptual model of the 4D Aetheric solution to the photoelectric effect
Current Saturation
There is a limitation to the amount of current that can be generated by the photoelectric effect. This is termed the saturation point, and is traditionally thought to be due to the fact that once the light frequency is increased by a certain degree, every photon will exhibit enough energy to produce a photoelectron. Therefore, increasing the voltage will have resulted in no further increase in the amount of current produced.
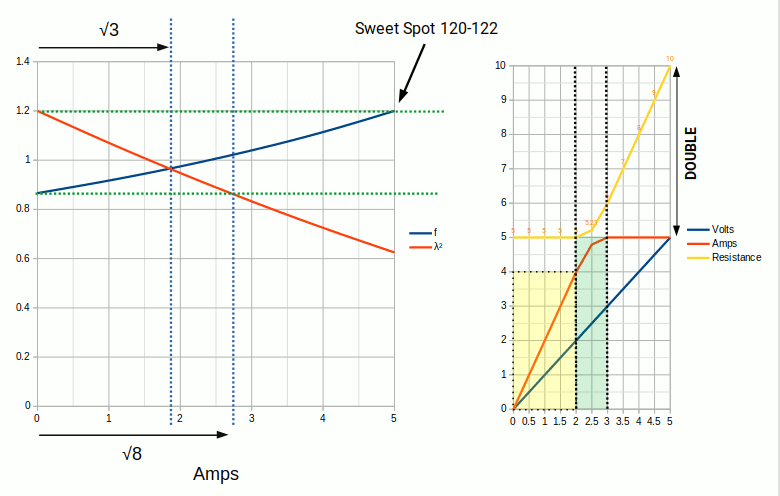
An alternative way of perceiving this is that once the current obtains its saturation level, any increase in voltage will equate to a proportional increase in resistance. This limits the curvature to a range of +3 Volts, which will produce up to 5 mA with a light intensity of 10. When we examine the curvature of electrical flow, we find that the emission of photoelectrons begins to exhibit an immediate increase in relationship to the applied voltage. This remains in direct proportion to the current produced until about +2 volts over the initial starting point. The final volt, after this, sees the current dramatically fall until it reaches saturation at around +3 volts. Altering the frequency does not change this curvature, it only moves it further toward the negative voltage side of the graph.
To find the two-thirds mark, we need to divide the starting wavelength of 2√3 by √1.5. We find that the curvature of the photoelectric effect is also directly related to the ‘sweet spot’ of between 1.2 and 1.22. After this point, the current relationship quickly begins to drop until saturation begins at +3V. This means the ratio changes from √1.5 to 2/√3 over this particular section of the curve. Therefore, at the +2V point, the EM wave produces a wavelength of √8. Just as the CMB has a frequency of √8, the point at which the curvature of the photoelectric effect begins to shift has a wavelength of √8.
As the wavelength and frequency are related by the speed of light (3), we find that when we multiply the CMB wavelength by 3, the result is also √8. In simple terms, this means that the point where the curvature start to change is three times the CMB wavelength, which is the ratio of the musical 5th.
The image above expresses this relationship to a square, side-length 1. What we see is that the inversion of the wavelength and frequency of an EM wave is simply the musical 5th. For those who are aware of the Planck-Einstein relation will be aware that photon energy is directly proportional to frequency and so inversely proportional to wavelength. This simple example shows how this nature is related to the musical 5th.
Next, we can examine the relationship between voltage and current by drawing two right-angled triangles that closely approximate the photoelectric effect curvature. The ratio of amps to volts is in a 2:1 ratio, which defines a triangle with a base of 2 and altitude of 4. What we notice is that this triangle has exactly the same dimensions as the one used in the Sandreckoner Diagram to square the circle. The final part of the curvature forms an almost perfect quarter arc, which can be wrapped around a square with a side-length of one. This gives the arc a distance of ½π.
This means that as the wavelength of 2√3 is divided by √1.5, the curvature suddenly changes from a 2:1 to a 1:1 ratio, forming a near perfect curvature as it reaches saturation point. This might seem like a strange geometric coincidence, but there is a slightly deeper significance to this nature.
We can plot a graph of frequency against wavelength squared. What we find is that as the wavelength decreases, the frequency increases proportionally. Once the value of 5 amps is reached, the frequency now holds the same value as the starting wavelength, which just happens to be the sweet spot between 120-122 nm. Furthermore, we can see that the square of the wavelength falls to the initial value of the frequency, the value for amps will equal √8.
We can also plot the value for the resistance for the circuit against the voltage and current. As the 1:2 ratio is maintained up to 2V and 4mA, the resistance maintains a proportional relationship of 5 Ohms. Between 2-3 Volts, we see a sharp curvature, after which Amps changes places with resistance to hold a consistent value of 5 mA. This means that as the voltage increases, the ratio to resistance now becomes 1:2, and amps remain at its saturation value of 5 mA.
In our geometric solution to the Reimann Hypothesis, we suggest that the process of analytic continuation produces the non-trivial zeros as the 0.5 mark, termed the critical strip, due to the folding of numerical space. Whilst all the non-trivial zero are found on the 0.5 mark, all trivial zeros will be found at -2. Similarly, the perfect ratio of ½ between amp and voltage, which changes to volts to resistance, is maintained by the same geometric principle. What this suggests is that the reason for the limitation of the saturation current is due to the folding of space, which switches the values for current and resistance, moving through the quarter arc of the circle, as it maintains a 1:2 ratio. In 4D Maths, this is represented by the collapsing of the infinite set of numbers into the horizontal plane to produce a line that is divided into 2. In the process of analytic continuation, this is achieved by swapping the complex numbers in the positive plane, with those in the negative plain, which compresses the infinite set of real numbers into a point around the number 1.
future ramifications
In this article, we have expressed a new solution to the photoelectric effect. As this process lies at the heart of modern quantum theory, it begins to bring into question the traditional scientific view of reality. The implications are quite profound for the future of technological development, and even humanity itself. For the process of turning sunlight into electrical energy now takes on a completely different perspective.
This new view explains many of the cutting-edge discoveries that are being pioneered in the field of sustainable energy. Solar cells coated with a thin film of magnesium are being shown to produce an increase in efficiency. Magnesium and sodium batteries, that are cheap and easy to produce, are now being explored as a sustainable alternative to toxic lithium-ion.
As our civilisation has become completely dependent on electricity, the need to develop cleaner and more sustainable solutions to energy generation has become evermore important. We believe that the theory of the 4D Aether offers a milestone turning point that will help us achieve our sustainable aims. Armed with a solid scientific theory of electrical generation by extracting energy from the vacuum, we pave the way for a sustainable zero emission society, where energy abundance becomes more readily available for all.
THE
Conclusion
How are the Photoelectrons created?
The emission of photoelectrons is triggered by specific wavelengths of light that resonate with the 4D Aether at the surface level of the material. This occurs at a point when the circular waves come into resonance with a square area of space. The result is that energy is extracted from the vacuum of space. The cosmic microwave background can no longer be seen as a relic of the Big Bang, as its frequency and wavelength are found to exhibit a strong correlation to the frequencies that produce the photoelectric effect.
What does this mean for the future?
The 4D Aether theory holds the key to unlocking the nature of extracting energy from the vacuum of space. Once we begin to recognise that the cosmic microwave background is in fact the Aether, then new ways to perfect the extraction of the vast amounts of energy stored in the level of Quantum Foam can be developed. The suggestion is that this will be enhanced by tuning our circuit design to specific resonant frequencies, which will help to improve the efficiency of solar cells and rechargeable batteries.
However, the theory presented will also have a profound effect on our scientific notion of the universe. As quantum mechanics is based on the wave-particle paradox, the 4D Aether suddenly resolves the mysteries of the quantum world. This actually makes sense of Max Planck’s original idea that light acts like oscillating strings. It also opens the door for a deeper understanding of the correlation between matter, energy, music and geometry, and offers a new mathematical system in aid of our future investigation of the subject.
Carry On Learning
This article is part of our new theory on the 4D AETHER. Browse more interesting post from the list below.


What is Quantum Foam? A 4D perspective
The idea of Quantum Foam suggests that at the smallest scales of reality, tiny energy fluctuation are present. These virtual particles are key to understanding the universe, yet seem to violate the laws of nature.
The 4D Electron Cloud
The electron cloud is normally thought about in terms of the probability of the particle. However, our notion of a 4D Aether dispenses with this notion, returning a sense of normally to atomic physics.

What is Ultraviolet Catastrophe? A harmonic solution to the Planck Constant.
When the classical interpretation of electromagnetism failed observational measurements of black body radiation, the Planck constant offer a solution to the Ultraviolet Catastrophe that suggested light was quantised into packets. However, an alternative solution can also be found in the nature of harmonic resonance.

Join the
Da Vinci School
Sign up for our Da Vinci School to access free online courses and explore the fascinating world of compass construction. Simplify complex knowledge through creative learning and make beautiful art!
YOUR QUESTIONS ANSWERED
Got a Question? Then leave a comment below.

Question?
I noticed that the CMB wavelength is at the millimetre scale, whereas the EM waves that produce the photoelectric effect are at the nanometre scale. How do you reconcile this difference?
ANSWER?
The CMB is qualified over a meter of space, whereas light waves are qualified in the photoelectric effect as a point particle (photon). There are 100,000 nanometres to a millimetre. The cube root of 100,000 is equal to 100, which is the scale of the EM light wave that produce the photoelectric effect.

Question?
When I checked the radius of the atoms, I get a different result to the sizes you publish in the article. Magnesium has a radius of 173 pm, whereas Argon is said to be larger with a radius of 188. How do you explain your results?
ANSWER?
When examining the radius of the atom, there are mathematical calculations based on present theory, then there are the measurements that are found through practical experiments. The figures you quote are from the Van Der Waal radius, which is a calculated value. The figures we quote are derived from physical observation. You can find out more about this by checking a data page, which lists these differences.