Principles of solid Geometry
1: A solid is that which has length, breadth, and depth.
2: A face of a solid is a surface.
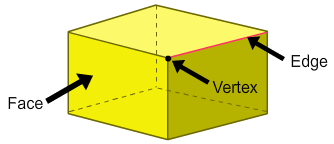
Lines and Planes
3: A straight line is at right angles to a plane when it makes right angles with all the straight lines which meet it and are in the plane.
4: A plane is at right angles to a plane when the straight lines drawn in one of the planes at right angles to the intersection of the planes are at right angles to the remaining plane.
5: Assuming a perpendicular drawn from the end of the straight line which is elevated above the plane to the plane, and a straight line joined from the point thus arising to the end of the straight line which is in the plane, the inclination of a straight line to a plane is the angle contained by the straight line so drawn and the straight line standing up.
6: The inclination of a plane to a plane is the acute angle contained by the straight lines drawn at right angles to the intersection at the same point, one in each of the planes.
7: A plane is said to be similarly inclined to a plane as another is to another when the said angles of the inclinations equal one another.
8: Parallel planes are those which do not meet.
3D Solids
9: Similar solid figures are those contained by similar planes, equal in multitude.
10: A solid angle is the inclination constituted by more than two lines which meet one another and are not in the same surface, towards all the lines, that is, a solid angle is that which is contained by more than two plane angles which are not in the same plane and are constructed to one point.
11: A pyramid is a solid figure contained by planes, which is constructed from one plane to one point.
12: A prism is a solid figure contained by planes two of which, namely those which are opposite, are equal, similar, and parallel, while the rest are parallelograms.
Spheres
13: When a semicircle with fixed diameter is carried round and restored again to the same position from which it began to be moved, the figure so comprehended is a sphere.
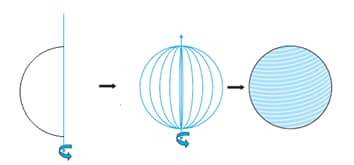
14: The axis of the sphere is the straight line which remains fixed and about which the semicircle is turned.
15: The centre of the sphere is the same as that of the semicircle.
16: A diameter of the sphere is any straight line drawn through the centre and terminated in both directions by the surface of the sphere.
Cones
17: When a right triangle with one side of those about the right angle remains fixed is carried round and restored again to the same position from which it began to be moved, the figure so comprehended is a cone. And, if the straight line which remains fixed equals the remaining side about the right angle which is carried round, the cone will be right-angled; if less, obtuse-angled; and if greater, acute-angled.
18:The axis of the cone is the straight line which remains fixed and about which the triangle is turned.
19: And the base is the circle described by the straight in which is carried round.
Cylinders
20: When a rectangular parallelogram with one side of those about the right angle remains fixed is carried round and restored again to the same position from which it began to be moved, the figure so comprehended is a cylinder.
21: The axis of the cylinder is the straight line which remains fixed and about which the parallelogram is turned.
22: And the bases are the circles described by the two sides opposite to one another, which are carried round.
23: Similar cones and cylinders are those in which the axes and the diameters of the bases are proportional.
Platonic Solids
24: A cube is a solid figure contained by six equal squares.
25: An octahedron is a solid figure contained by eight equal and equilateral triangles.
26: An tetrahedron is a solid figure contained by four equal and equilateral triangles.
27: A dodecahedron is a solid figure contained by twelve equal, equilateral and equiangular pentagons.
28: An icosahedron is a solid figure contained by twenty equal and equilateral triangles.
Explore more
geometric principles
This post is part of our Guide to Euclidean Geometry. You can explore more or return to the index page to follow the series in sequential order.
Up Next
The Sphere
Welcome, to this online guide to Euclidean Geometry, produced by In2infinity, and written by Sunil…
