In2infinity
Welcome to In2Infinity – a portal that transcends the boundaries between science, art, mathematics, and music. Our platform offers unique solutions to some of nature’s most fascinating secrets, presenting a groundbreaking theory of the universe rooted in geometry and a novel mathematical system.
Meet The Founders
In2infinity is based on the discoveries of Colin Power and was founded in conjunction with Dr. Heike Bielek.
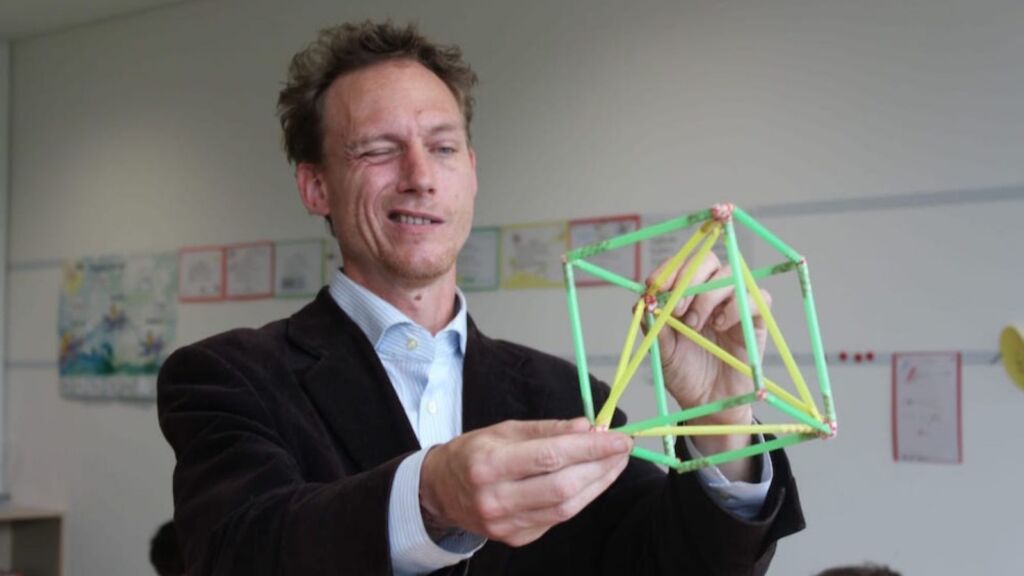
COLIN POWER
MATHEMATICS, MUSIC & EDUCATION
In the world of mathematics and geometry, one name stands out – Colin Power. With a lifetime of extraordinary experiences fuelling his passion, In2Infinity was founded in 2015 to share the groundbreaking discoveries of this mathematical maverick.
Colin’s relentless dedication has unearthed revelations with profound implications across disciplines like quantum sciences and biology. In2Infinity stands testament to his lifelong commitment to unravelling the beauty and intricacies of fundamental principles.
Dr. Heike Bielek
Biology, Art, Yoga & EDUCATION
Dr. Heike Bielek’s journey, rooted in biosciences, took a transformative turn during travels to India, where she immersed herself in the geometric matrix of reality through the lens of Yoga and symbols.
Driven by Colin’s discoveries, Dr. Bielek joined forces, leveraging her academic background, passion for art, and music to make geometric knowledge accessible. Her interdisciplinary approach employs engaging teaching styles, connecting with students across scientific and philosophical disciplines.
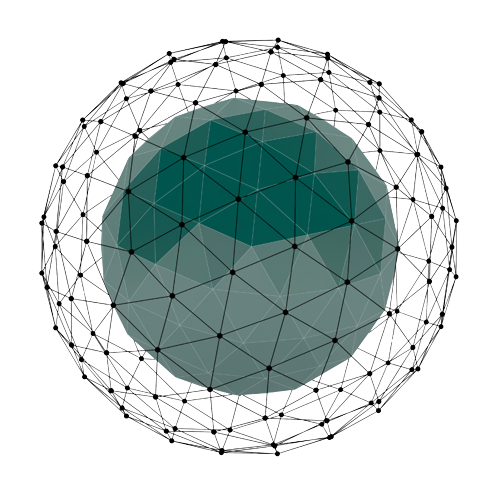
The Geometric Theory
Envision a theory that not only unravels the mysteries of the physical world but also illuminates spiritual and religious teachings, all rooted in the principles of geometry and a unique mathematical system. In2Infinity explores the laws of mathematics and geometry that govern our reality, providing a holistic approach to understanding the universe. From solving intricate mathematical problems to introducing a novel geometric model of the atom, In2Infinity reinterprets scientific discoveries through the lens of higher dimensional Euclidean geometry. Revealing a captivating fractal-holographic mechanism shaping the cosmos, In2Infinity serves as a portal to a vast universe of knowledge, welcoming both experts and enthusiasts to explore uncharted realms of understanding.
No one likes a smart ass. And so it has been my experience that if you do resolve the answer to life, the universe, and everything, you are better off not going around shouting about it. Though to be fair, circumstances also play a role. For me, a travelling musician, with a compass, ruler, and $1 calculator (from Indonesia as I needed the 16 digits); to come up with a new Geometric theory of the Universe in just 28 days, whilst confined in a beach hut without internet? It was going to be hard for the average scientist to give any credence.
As a wise man once said, ‘there is nothing new under the sun.’ a sentiment we should bear in mind when making claims over our understanding of the Universe. In fact, the Geometry used to be at the centre of our scientific methodology. As our ability to use technology to measure the fabric of reality has increased, the significance of Geometry has decreed in the eyes of the masses. Yet, geometric principles are still used to qualify the universe. When we extrapolate these into higher dimensions, we find a model emerges that has yet to be considered by the mainstream scientific community.
The Geometric Universe theory is unique as it is the first to explain scientific findings from dimensional space higher than the 3D world, without resorting to speculative theories about how multidimensional space is constructed. This in turn brings a deeper comprehension to the spiritual and religious concepts prevalent throughout the world. The result is a new approach to explaining the nature of life, the universe, and beyond.
Support us on Patreon!
Join our monthly webinars, where we discuss the developments of our geometric theory by supporting us on Patreon. Your contributions and engagement will help us explore our findings more deeply.
